- 表紙
- まとめ
- Dance
- 定義
- 具体的な値
- 注意点
数学のまとめ
「三角比」とは
角度を三角形の辺の長さの比を使って表現した値のこと。
鋭角の三角比の定義
$C = 90^{\circ}$ の直角三角形 $\mathrm{ABC}$ において, $A=\theta$ ならば, 正弦
$\displaystyle \sin \theta = \frac{a}{c}$
, 余弦
$\displaystyle \cos \theta = \frac{b}{c}$
, 正接
$\displaystyle \tan \theta = \frac{a}{b}$
を定める.
B. 三角比の相互関係
① $\sin^2 \theta + \cos^2 \theta = 1$
② $\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$
③ $\displaystyle 1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}$
三角比の拡張
$xy$ 平面の単位円上の点 $\mathrm{P}$ が $\angle \mathrm{POX} = \theta$ であるとき, $0^{\circ} \leqq \theta \leqq 180^{\circ}$ の三角比は,
$\sin \theta = y$
,
$\cos \theta= x$
,
$\tan \theta = y/x$
で定義される. なお, $\mathrm{O}(0,0)$, $\mathrm{X}(1,0)$ とする.
C. 余角と補角に関する三角比の関係
① $\sin (90^{\circ} - \theta) = \cos \theta$
② $\cos (90^{\circ} - \theta) = \sin \theta$
③ $\displaystyle \tan (90^{\circ} - \theta) = \frac{1}{\tan \theta}$
❶ $\sin (180^{\circ} - \theta) = \sin \theta$
❷ $\cos (180^{\circ} - \theta) = -\cos \theta$
❸ $\displaystyle \tan (180^{\circ} - \theta) =- \tan \theta$
ポイント解説
定義
直角三角形の図は次の通り:

例
三角定規の直角三角形
| $\theta$ | $30^{\circ}$ | $45^{\circ}$ | $60^{\circ}$ |
| $\sin \theta$ | $\frac{1}{2}$ | $\frac{\sqrt{2}}{2}$ | $\frac{\sqrt{3}}{2}$ |
| $\cos \theta$ | $\frac{\sqrt{3}}{2}$ | $\frac{\sqrt{2}}{2}$ | $\frac{1}{2}$ |
| $\tan \theta$ | $\frac{1}{\sqrt{3}}$ | $1$ | $\sqrt{3}$ |
拡張
図は次の通り:

他の三角比
上の三角比の逆数に対応:
- 余割: $\csc \theta =c/a$
- 正割: $\sec \theta = c/b$
- 余接: $\cot \theta = a/b$
定義をDANCEで暗記
こんにちは。早速ですが、三角比の単元の一番最初の公式をすぐに覚えられるでしょうか?もしくは、すぐに教えられるでしょうか?
三角比の定義の公式は、昔の人が勝手に決めたことですから、覚え込んでしまわなければいけません。
公式が覚えられないーという方々に対して、頭で覚えるのではなく、身体で覚え込んで貰う方法を生み出しました!
※本当に数学が苦手な生徒への指導方法伝授の記事です。
目次
三角比の定義の公式について
三角比の定義の公式を確認しましょう。
直角三角形 ABC で、$\sin A$ や $\cos A$, $\tan A$ というものを決めます。
それぞれの角度に対応する辺の長さを $a$, $b$, $c$ とおきます。
次の画像を参考にしてください。

※ 直角三角形で対応する角度( $A$ )を左側、直角( $C$ )を右側におきます。
このとき、三角比の定義は次の通りです。
- $\displaystyle \sin A = \frac{a}{c}$
- $\displaystyle \cos A = \frac{b}{c}$
- $\displaystyle \tan A = \frac{a}{b}$
これらの式の分数は、特に、法則があるという訳ではありません。
したがって、この3つの分数は覚えなければいけません。また、実際に計算を行うときには文字ではなく数字ですので、直角三角形内の「位置」として覚える必要があります。
※すぐに暗記できると良いですが、なかなか暗記できない人もいるのが実情です。
三角比の定義の公式の暗記方法
三角比の定義の公式を覚える方法、覚えさせる方法を考えましょう。
三角比の定義式の有名な暗記方法
3つの三角比の定義の公式を覚える有名な方法を紹介します。
英単語の頭文字の筆記体で覚えるという方法です。
- サインは、sin なので、イニシャルの「s」の筆記体として覚える
- コサインは、cos なので、イニシャルの「c」の筆記体として覚える
- タンジェントは、tan なので、イニシャルの「t」の筆記体として覚える
しかし、この方法は、筆記体に慣れていない人にとっては、覚えやすいものではありません
したがって、英語に嫌悪感を抱く人にとっては、余計に混乱してしまう結果となります。
この困ったことを解消するために、身体で覚えてもらうダンスを考えました!
三角比の公式暗記ダンス
ダンスの方法は簡単です。公式の通りに、腕を動かすだけです。腕を動かした通りに、分数を書くことを行ないます。
gif動画を作りました。見てください!

このようにして貰うと、授業は楽しいし、身体で三角比を覚えることができます!
本当に、数学が苦手な生徒用の指導の方法です!
三角比の公式暗記ダンスの解説
公式暗記ダンスの核となる部分を解説します。
動画内の棒人間は向こう(画面の奥)を向いている設定です!
このダンスを行うときの最も大切なことは、直角三角形はいつでも、対象の角(現在は $A$)が左にあり、直角(現在は $C$ )が右にあるようにしておくことです。
$\sin A$ のふりつけ
まず、左腕を直角三角形の上の部分(角B)に沿うように回してあげます。これがサインの暗記方法です。

$c$ に沿った後に、$a$ に沿うように腕を動かすので、
$$\frac{a}{c}$$
と分数を作ってください。これが、$\sin A$ です。
したがって、$\displaystyle \sin A = \frac{a}{c}$ です。
$\cos A$ のふりつけ
次に、左腕を直角三角形の左の部分(角A)に沿うように回してあげます。これがコサインの暗記方法です。

$c$ に沿った後に、$b$ に沿うように腕を動かすので、
$$\frac{b}{c}$$
と分数を作ってください。これが、$\cos A$ です。
したがって、$\displaystyle \cos A = \frac{b}{c}$ です。
$\tan A$ のふりつけ
最後に、右腕を直角三角形の右の部分(角C)に沿うように回してあげます。これがタンジェントの暗記方法です。

$b$ に沿った後に、$a$ に沿うように腕を動かすので、
$$\frac{a}{b}$$
と分数を作ってください。これが、$\tan A$ です。
したがって、$\displaystyle \tan A = \frac{a}{b}$ です。
以上で、ダンスの順番と、三角比の分数の作り方が分かりました。
三角比の定義公式の暗記方法まとめ
今回のダンスは、英語の筆記体では、すぐに覚えられないという方に対しての暗記手段として活用することができます。
このダンスがあまり役に立つ機会はないかもしれませんし、授業の時に前で実践することは恥ずかしいですが、一度やってみることはオススメします。
数学の指導は、たくさんの知識をどれだけ整理して、各生徒の中に体系づけることができるかが大切になります。
その際に、一つ一つの知識に印象付けを行うことは大事な要素となります。今回のダンスは、印象付けにはモッテコイの方法となります。

どうぞ、一度おどってみてください!
ここまで、お読みいただきありがとうございます。
定義式の理解
三角比の定義の式の本質を理解するための解説を書きました。
三角比の定義は、相似な直角三角形同士で無関係に式の値が定まることが重要ポイントです。
三角比の定義とは
三角比の定義の公式の確認
三角比の定義を確認しておきます。
直角三角形ABCの角度の三角比(3つ)とは、次の数式で定まる値のことです。(角 $C$ が直角とする。)
- $\displaystyle \sin A = \frac{c}{a}$
- $\displaystyle \cos A = \frac{c}{b}$
- $\displaystyle \tan A = \frac{b}{a}$

それぞれの角に対応する辺の長さを $a$, $b$, $c$ として、三角比の定義の数式に代入することでそれぞれの三角比の値を定めることができます。
三角比の定義の疑問❓
定義の解説は以上ですが、何も疑問に感じないでしょうか?
これから話を簡単にするために、$\tan 60^{\circ}$ で説明します。(tan が最も存在感が薄いみたいですので。)サインとコサインについても話は同じです。
定義式の計算手順を整理しましょう。
- 指定された角度を左側、直角を右側にして、直角三角形を置く。
- 辺の長さを2つ選び、分母(底辺の長さ)と分子(高さの長さ)に置く。
- そして、角度 $A$ の前に、$\tan$ の記号を付ける。
この3つの手順に疑問を持って欲しい箇所はありません。手順以前のところに疑問を抱いて欲しいです!
どんな直角三角形を考えましたか?
例えば、$\tan 60^{\circ}$ を求める場合、$A=60^{\circ}$, $C=90^{\circ}$ ( $B=30^{\circ}$ )の直角三角形を考えます。しかし、この条件を満たす直角三角形は沢山あります。相似な三角形の分だけ沢山あります。
抱いてほしい疑問とは、次の疑問です。
大きさの違う他の相似な直角三角形で三角比を計算すると、$\tan 60^{\circ}$ が違う値になってしまうのではないか?
三角比の定義の本質
疑問に答える形で、三角比の定義の本質を解説します。
相似な三角形の復習
相似の定義を確認しましょう。
三角形に限らず、2つの図形が相似な関係であるとは、一方の図形を拡大もしくは縮小することで合同な関係になることです。
相似とは「大きさが違うだけで形が一緒」ということですね。
ここから図形を三角形に限定します。中学校のときに、2つの三角形が相似であるための相似条件を習いました。覚えていますか?
- 3組の辺の長さの比が全て等しい。
- 2組の辺の長さの比と、その間の角の大きさがそれぞれ等しい。
- 2組の角の大きさがそれぞれ等しい。
そして、
『相似条件が条件が成り立つ $\Longrightarrow$ 2つの三角形は相似である』
です。しかし、この逆が(もちろん)成り立ちます。
『2つの三角形が相似である $\Longrightarrow$ 相似条件が成り立つ』
2つの三角形が相似であれば相似条件で言われていることが成り立ちます。
相似でも三角比の定義の値が一致
確かめたいことは、どちらの三角形で三角比を計算しても同じ値になるかどうかです!
2つの三角形 ABC(辺のながさが $a$, $b$, $c$)と A'B'C'(辺の長さが $a'$, $b'$, $c'$) が相似であって、相似比が $k$ だとします。
特に相似条件の①の条件に注目すると『2つの相似な三角形の対応する辺の長さの比は全て等しい』が成り立ちます。
次の関係が成り立ちます。
三角形ABCで $\tan A$ を計算
三角形ABCと三角形A'B'C'のそれぞれで三角比を計算してみます。(タンジェントで計算してみます。)
三角形ABCで計算すると、そのまま $\displaystyle \tan A = \frac{b}{a}$ です。
三角形A'B'C'で $\tan A'$ を計算
次に、三角形A'B'C'で計算してみましょう。$b' = kb$, $c' = kc$ です。
どちらの三角形で計算しても、$\tan A$ も $\tan A'$ も $\displaystyle \frac{b}{c} = \frac{b'}{c'}$ という同じ値になりました。
三角形が違っても、相似である限り、三角比の定義の値は違う数値にならないことが確認できました。他の三角比のサインとコサインでも同様です。
これが、三角比の定義式が分数であることの本質です。
相似な三角形と三角比の定義の具体例
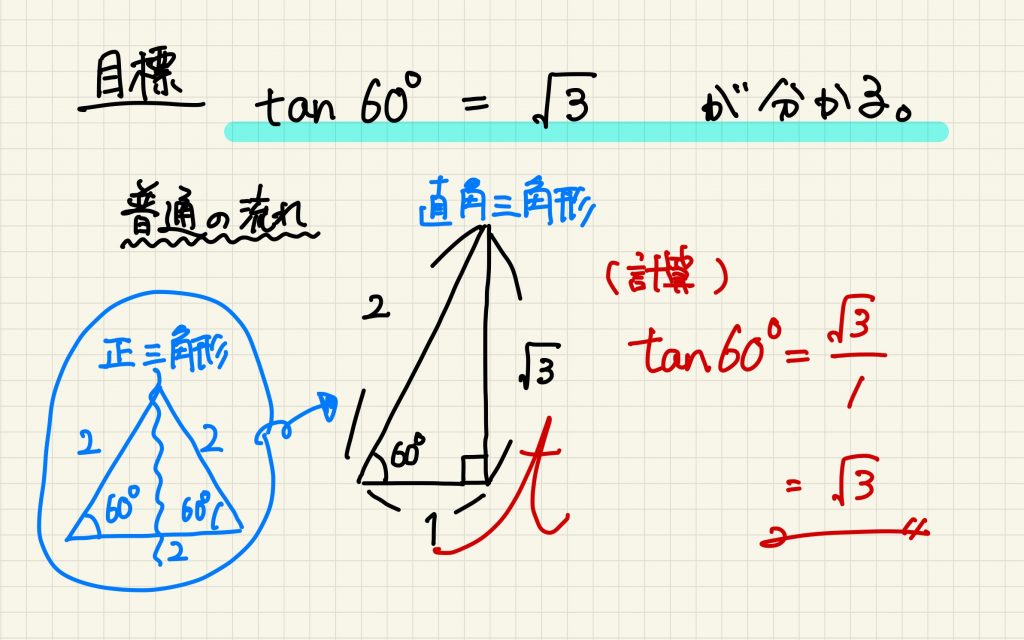
イラストを2枚作成したので、$\tan 60^{\circ} = \sqrt{3}$ であることが、相似な直角三角形に依らず定まることを確かめましょう。
1枚目のイラストは、$\tan 60^{\circ}$ を求めるための直角三角形が正三角形を半分にして出来た形であることと、定義の計算をしています。
2枚目のイラストは、大きさの違う2つの相似な三角形( $1:2$ の相似比 )があったときに、$\tan 60^{\circ}$ の値が一致することを具体的な数値で確かめています。
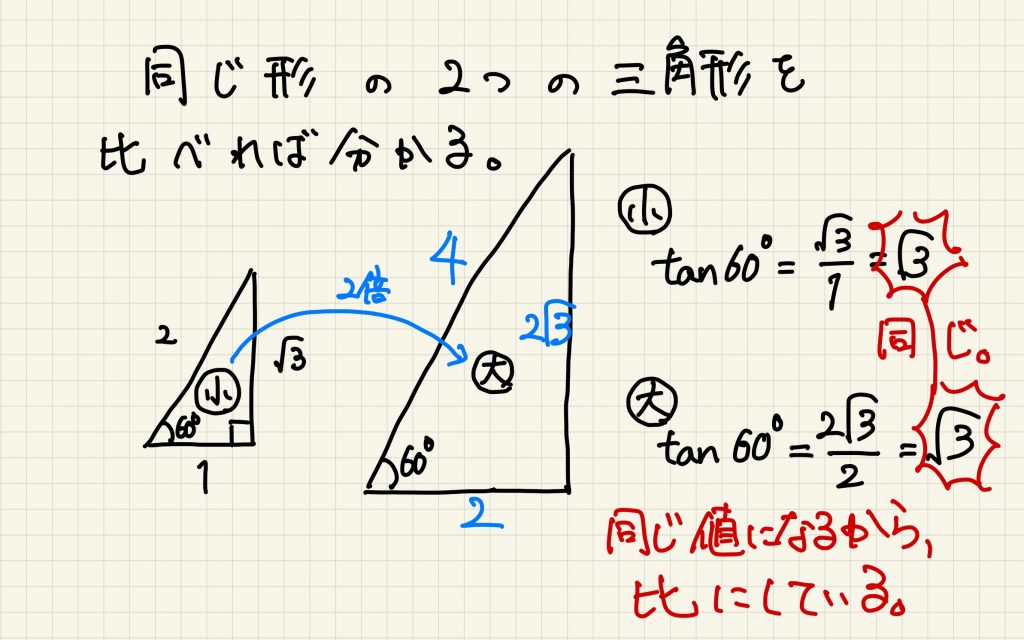
サインやコサインも含めて、他の三角比においても、直角三角形の大きさに依らず、三角比の値が決まることを確かめてみてください。
三角比の定義の本質を理解するQ&A
今までの内容が理解できていれば、よく挙がる疑問に答えることができます!
-
三角比の公式って、なんで分数の形(複雑な形)をしているの?
-
角の大きさと辺の長さを繋げるための数式としては、分数の形が最も合理的(かつシンプル)だからです。
つまり、$\sin A = a$ のような式だと、考える直角三角形に依って値がバラバラになってしまいます。しかし、辺の長さを比にすることで、相似比の違いは、約分という計算によって気にしなくてよいことになります。
三角比の定義は複雑な形をしているように見えて、角度と辺の長さを結びつける最も合理的な式なのです!角度と辺の長さが、分数という一工夫だけで結びつけられるています。見方を変えれば、非常にシンプルに表現できている式だと感じることができます。
-
相似な三角形に依らず決まることは分かったけど、それって何かの役に立つの?
-
めちゃくちゃ良い質問ですね。相似な三角形に関係なく三角比が決まることを解説しました。言い換えると、相似な三角形であれば、どの三角形で三角比を計算しても良い、ということです。
現実世界で現れる大きな直角三角形(木の高さを測るときとかに出くわす)の辺の長さや角度を調べるときに、もっと小さい、手のひらに収まる(相似な)三角形で計算を代用できる、ということになります。
めちゃくちゃ大きな対象であって計算が手に負えないように見えても、取り扱い可能な対象に代用できるということです。
1つ目の質問は、生徒からよく聞きます。2つ目の質問は、未だかつて生徒から出たことはありませんが、大切なことです。
三角比の定義は、角の大きさと辺の長さ(の比)を結びつける画期的な数式である。定義の値を決めるためには、直角三角形を一つ用意する必要があるが、どの(相似な)直角三角形を用意しても問題なく、同じ値が定まる。辺の長さを比(分数)としていることは複雑に見えるけれども本質である。
以上で、角の大きさと辺の長さ(の比)を結びつける三角比の定義を理解することができました。

三角比の定義の数式を導入することで、非常に大きな三角形であっても、小さいな三角形であっても、取り扱い可能な(相似な)三角形にすることで扱うことができる素晴らしさがあります。
ここまで、お読みいただき、ありがとうございます。
有名角の三角比の値
平方根と分数で表すことができる有名角の三角比の値を計算して、整理しました。
目次
三角比の表
教科書の巻末にある三角比の表をExcelで作りました。

有名角の三角比
鋭角の有名角($30^{\circ}$, $45^{\circ}$, $60^{\circ}$)
| $\theta$ | $30^{\circ}$ | $45^{\circ}$ | $60^{\circ}$ |
| $\sin \theta$ | $\displaystyle \frac{1}{2}$ | $\displaystyle \frac{\sqrt{2}}{2}$ | $\displaystyle \frac{\sqrt{3}}{2}$ |
| $\cos \theta$ | $\displaystyle \frac{\sqrt{3}}{2}$ | $\displaystyle \frac{\sqrt{2}}{2}$ | $\displaystyle \frac{1}{2}$ |
| $\tan \theta$ | $\displaystyle \frac{1}{\sqrt{3}}$ | $1$ | $\sqrt{3}$ |
$0^{\circ}$ 〜 $180^{\circ}$ までの有名角
| $\theta$ | $0^{\circ}$ | $30^{\circ}$ | $45^{\circ}$ | $60^{\circ}$ | $90^{\circ}$ | $120^{\circ}$ | $135^{\circ}$ | $150^{\circ}$ | $180^{\circ}$ |
| $\sin \theta$ | $0$ | $\displaystyle \frac{1}{2}$ | $\displaystyle \frac{\sqrt{2}}{2}$ | $\displaystyle \frac{\sqrt{3}}{2}$ | $1$ | $\displaystyle \frac{\sqrt{3}}{2}$ | $\displaystyle \frac{\sqrt{2}}{2}$ | $\displaystyle \frac{1}{2}$ | $0$ |
| $\cos \theta$ | $1$ | $\displaystyle \frac{\sqrt{3}}{2}$ | $\displaystyle \frac{\sqrt{2}}{2}$ | $\displaystyle \frac{1}{2}$ | $0$ | $\displaystyle -\frac{1}{2}$ | $\displaystyle -\frac{\sqrt{2}}{2}$ | $\displaystyle -\frac{\sqrt{3}}{2}$ | $-1$ |
| $\tan \theta$ | $0$ | $\displaystyle \frac{1}{\sqrt{3}}$ | $1$ | $\sqrt{3}$ | × | $-\sqrt{3}$ | $-1$ | $\displaystyle \frac{1}{\sqrt{3}}$ | $0$ |
$15^{\circ}$ と $18^{\circ}$ の倍数の三角比
$15^{\circ}$, $18^{\circ}$, $36^{\circ}$
$\theta = 15^{\circ}$
$\displaystyle \sin 15^{\circ} = \frac{\sqrt{6}-\sqrt{2}}{4}$
$\displaystyle \cos 15^{\circ} = \frac{\sqrt{6}+\sqrt{2}}{4}$
$\displaystyle \tan 15^{\circ} = 2-\sqrt{3}$
$\theta = 18^{\circ}$
$\displaystyle \sin 18^{\circ} = \frac{\sqrt{5}-1}{4}$
$\displaystyle \cos 18^{\circ} = \frac{\sqrt{10 + 2\sqrt{5}}}{4}$
$\displaystyle \tan 18^{\circ} = \frac{1}{\sqrt{5+2\sqrt{5}}}$
$\theta = 36^{\circ}$
$\displaystyle \sin 36^{\circ} = \frac{\sqrt{10 - 2\sqrt{5}}}{4}$
$\displaystyle \cos 36^{\circ} = \frac{\sqrt{5}+1}{4}$
$\displaystyle \tan 36^{\circ} = \sqrt{5-2\sqrt{5}}$
$54^{\circ}$, $72^{\circ}$, $75^{\circ}$
$\theta = 54^{\circ}$
$\displaystyle \sin 54^{\circ} = \frac{\sqrt{5}+1}{4}$
$\displaystyle \cos 54^{\circ} = \frac{\sqrt{10 - 2\sqrt{5}}}{4}$
$\displaystyle \tan 54^{\circ} = \frac{\sqrt{25+10\sqrt{5}}}{5}$
$\theta = 72^{\circ}$
$\displaystyle \sin 72^{\circ} = \frac{\sqrt{10 + 2\sqrt{5}}}{4}$
$\displaystyle \cos 72^{\circ} = \frac{\sqrt{5}-1}{4}$
$\displaystyle \tan 72^{\circ} = \sqrt{5+2\sqrt{5}}$
$\theta = 75^{\circ}$
$\displaystyle \sin 75^{\circ} = \frac{\sqrt{6}+\sqrt{2}}{4}$
$\displaystyle \cos 75^{\circ} = \frac{\sqrt{6}-\sqrt{2}}{4}$
$\displaystyle \tan 75^{\circ} = 2+\sqrt{3}$
記号と公式の注意点
三角比の単元のときの注意点リストを書きました。
特に、相互関係の公式に絞って書いています。
目次
三角比の相互関係の公式
三角比の相互関係の公式は、数学I(三角比)と数学Ⅱ(三角関数)の教科書を合わせると3回同じ式が出てきます。
- $\displaystyle \sin^2 \theta + \cos^2 \theta = 1$
- $\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$
- $\displaystyle \tan^2 \theta + 1 = \frac{1}{\cos^2 \theta}$
2乗の書き方(教えてもらえない時も)
三角比の2乗(平方)は、通常と書き方が違います。
$\sin A$ の平方のことを $\sin^2 A$ と表記します。$\sin A^2$ とは表記しません。
$$\sin^2 A =( \sin A )^2 = \sin A \times \sin A$$
$\sin$ に2乗を付ける理由は、$\sin A$ を2乗しているのか、$A$ を2乗しているのかを区別するためです。
具体的に見ると、違いがよく分かります。
$\sin^2 25^{\circ} = \sin 25^{\circ} \times \sin 25^{\circ}$ と、
$\sin 25^{\circ} \ {}^2 = \sin 625^{\circ}$ は違います。
シータについて(適当に書かない)
角度を表す記号でシータ $\theta$ が登場します。
シータ $\theta$ はギリシャ文字です。
アルファ $\alpha$ や ベータ $\beta$ の仲間です。(通常使用している、アルファベットの $a$ や $b$ は、ラテン文字(ローマ字)です。)
ギリシャの文字を利用しています。
変数 $\theta$ の意味(大事!)
三角比(三角関数)の変数である $\theta$ の意味は3通りあります。
正確な説明は授業であまりされないかもしれません。
鋭角の角度 $\theta$
鋭角とは $0^{\circ} < \theta < 90^{\circ}$ の角度のことです。
$\theta$ が鋭角のときは、対応する直角三角形があ流ので、三角形の一つの角度を使いやすいです。
$\theta$ ではなく、三角形の角度を表す $A$ を利用することが多いです。
$0 \sim 180$ 度の角度 $\theta$
0度から180度までの角度のときは、対応する直角三角形が考えられないので、三角形の頂点を表す $A$ などは利用できません。
そこで、$A$ などの記号よりも $\theta$ が好まれて使われます。
弧度としての長さ $\theta$
弧度法(円弧の弧長)のときは、$\theta$ は厳密には長さです。
長さの場合は、よく利用する変数である $x$ を変数として使うことも自然です。
だから、数学Ⅱの三角関数の単元では、$\sin \theta$ や $\sin x$ が両方出てきます。
三角比の相互関係の計算テクニック
公式の使い分け(最も大事!)
相互関係の公式を計算するときの注意点に移ります。
- $\displaystyle \sin^2 \theta + \cos^2 \theta = 1$
- $\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$
- $\displaystyle \tan^2 \theta + 1 = \frac{1}{\cos^2 \theta}$
公式の番号は、①②③を使います。
そもそも相互関係の公式は、1つの三角比の値が分かっているときに、他の三角比の値を求めるために利用することが大きな役割です。
既知の三角比を1列目に、求めたい三角比を1行目に書きました。
| 求める値→ | $\sin A$ | $\cos A$ | $\tan A$ |
| $\sin A$ | × | まず①を使う | 次に②を使う |
| $\cos A$ | まず①を使う | × | 次に②を使う |
| $\tan A$ | 次に②を使う | まず③を使う | × |
他には、$\cos A$ が分かっているときに、$\tan A$ だけを求める必要があります。この場合は、③の公式だけを利用しましょう。
公式の形の変形(計算しやすく)
相互関係の②の公式は、3つの形に変えられることが大切です。
特に、計算が苦手な方は、知っておいてください!
②の公式は、次の形でした。
$$\tan A = \frac{\sin A}{\cos A}$$
次の2つの式も同じ公式です。
$$\tan A = \sin A \div \cos A$$
$$\sin A = \tan A \times \cos A$$
$\tan A = \sin A \div \cos A$
公式の一つ目の変形パターンは、$\sin A$ と $\cos A$ から、 $\tan A$ を求める際に便利です。
公式の元の形のままでは、「分数ぶんの分数」になってしまうなることが多く、計算が苦手な人は間違えやすいです。
「分数 $ \div$ 分数」とすれば、後ろの分数をひっくり返して掛け算をすれば良いので、計算が容易になります。
$\sin A = \tan A \times \cos A$
公式の二つ目の変形パターンは、$\tan A$ と $\cos A$ から、$\sin A$ を求める際に便利です。
2つの三角比を掛けるだけで、$\sin A$ を求められるので考えやすいです。
以上、相互関係の公式②について、計算がしやすい表記を紹介しました。
その他の注意点
三角比の相互関係の公式は、慣れると機械的に計算できます。
しかし、初めて学ぶときには、ややこしい表記や計算が混在しています。
学ぶ方も、教える方も忘れずにしないといけないことを整理しました。
あと、今回は書きませんでしたが、苦手な方は、次の2点も知っておくと苦手が解消されると思います。
$\cos A$ が分数のときの $1/\cos^2 A$の計算
$\cos A$ が分数である場合が多いです。③の公式を利用するとき、$\displaystyle \frac{1}{\cos^2 A}$ の計算をどうするかは、苦手な方は知っておく必要があります。
平方根をとるときの三角比の正負
角度 $\theta$ の存在範囲によって、$\sin^2 A$ から2乗を外して $\pm \sin A$ にしたとき、正負のどちらを選ぶかを決める必要があります。
これらの2点は、今回は割愛しました。すみません。


